What is it about?
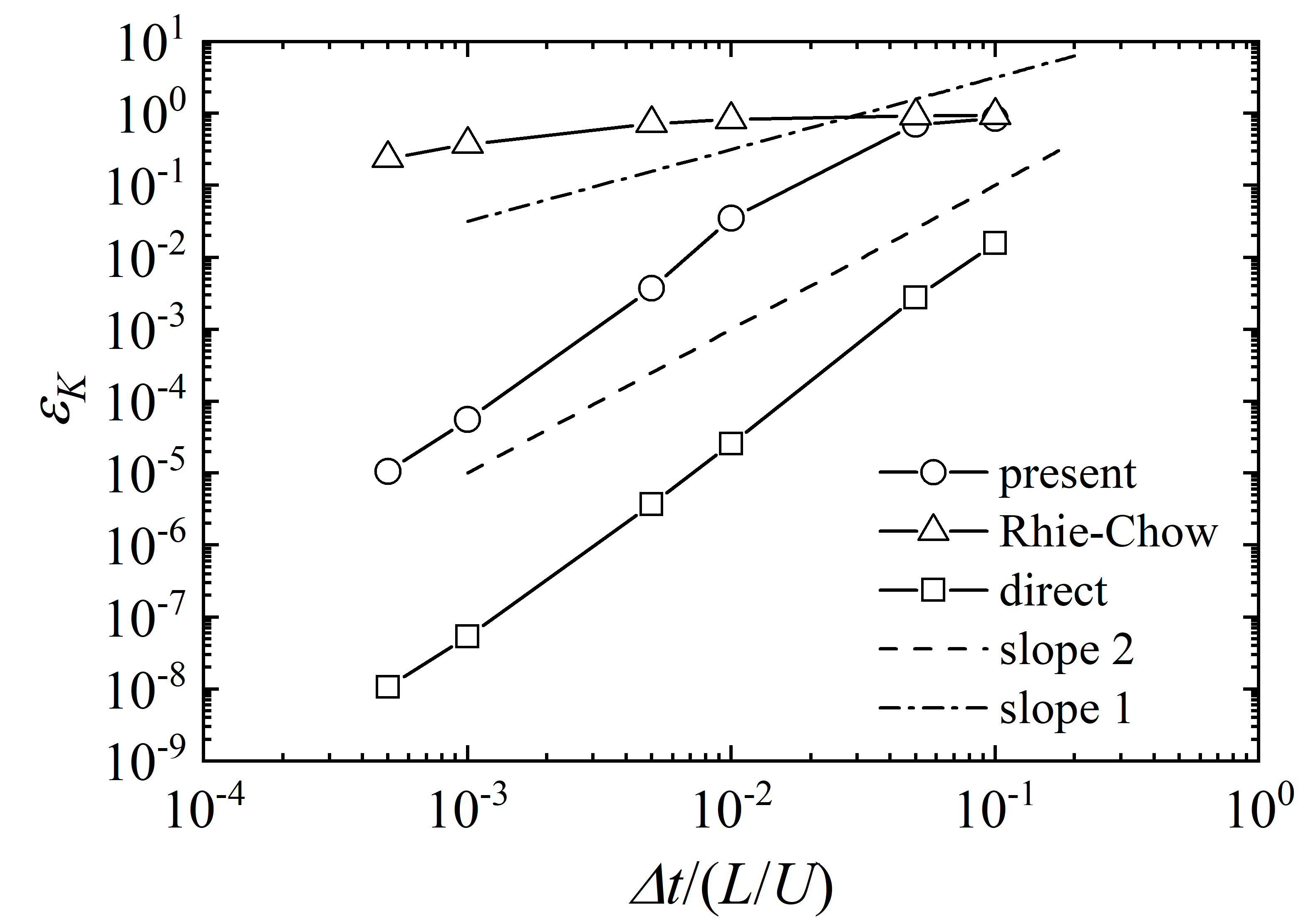
Numerical methods using staggered or collocated grids are commonly used to analyze incompressible flows. The collocation method utilizes the Rhie-Chow scheme to find the cell interface velocity through pressure-weighted interpolation. However, the energy conservation property and the accuracy of this interpolation method in unsteady flows have not yet been fully elucidated. This study constructs a finite difference scheme for incompressible fluids using a collocated grid in a general curvilinear coordinate system. The velocity at the cell interface is determined by weighted interpolation based on the pressure difference to prevent pressure oscillations. The Poisson equation for the pressure correction value is solved with the cross-derivative term omitted to improve calculation efficiency. Moreover, simultaneous relaxation of velocity and pressure is applied to improve convergence. Even without the cross-derivative term, calculations can be stably performed, and convergent solutions are obtained. In unsteady inviscid flow, the conservation of kinetic energy is excellent even in a non-orthogonal grid, and the calculation result has second-order accuracy in time. When analyzing viscous flow at a high Reynolds number, the error decreases compared to the Rhie-Chow interpolation method. The present numerical scheme improves calculation accuracy in unsteady flows and demonstrates the potential for applying this computational method to high Reynolds number flows through several analyses.
Featured Image

Photo by Andrei J Castanha on Unsplash
Why is it important?
The energy conservation property and the accuracy of the Rhie-Chow interpolation method in collocated grids have not yet been fully elucidated. Cross-derivative terms always appear in the fundamental equations when the collocation method is expanded to general curvilinear coordinate systems. When analyzing complicated three-dimensional flow fields, the increase in memory usage and computation time leads to inefficient computation. Therefore, it is necessary to construct a method that can perform stable and highly accurate calculations even if the cross terms are omitted. I built a finite difference scheme for incompressible flows in a general curvilinear coordinate system using a collocated grid and explored kinetic energy conservation properties and an efficient method for finding pressure. Moreover, I simultaneously relaxed the Poisson equation for the pressure correction value and the modified equations for velocity and pressure so that convergence did not deteriorate even if the cross terms were omitted. Using the computational method proposed in this study, I analyzed several flow fields and verified that the time discretization accuracy could be improved in unsteady flow fields.
Perspectives

The energy conservation properties of the Rhie-Chow interpolation method used in collocation methods have not been sufficiently clarified in unsteady flows. Additionally, a numerical method that can perform stable and highly accurate calculations, even if omitting the cross terms of the Poisson equation for pressure in a general curvilinear coordinate system, has not been proposed. This study constructed a finite difference scheme for incompressible fluids using a collocated grid in a general curvilinear coordinate system. It has been clarified that the present numerical scheme improves the calculation accuracy in unsteady flows. Therefore, this method can contribute to understanding coherent vortices and heat transport at high Reynolds number flows.
Professor Hideki Yanaoka
Iwate Daigaku
Read the Original
This page is a summary of: Energy-conserving finite difference scheme based on velocity interpolation applicable to unsteady flows using collocated grids, Numerical Heat Transfer Part B Fundamentals, June 2024, Taylor & Francis,
DOI: 10.1080/10407790.2024.2360045.
You can read the full text:
Contributors
The following have contributed to this page